Detail of Publication
| Text Language | English |
|---|---|
| Authors | Masayuki Miyamoto, Masakazu Iwamura and Koichi Kise |
| Title | A Quantum Algorithm for Minimum Steiner Tree Problem |
| Journal | Proc. 19th Asian Quantum Information Science Conference (AQIS2019) |
| Number of Pages | 2 pages |
| Location | Seoul, Korea |
| Reviewed or not | Reviewed |
| Presentation type | Poster |
| Month & Year | August 2019 |
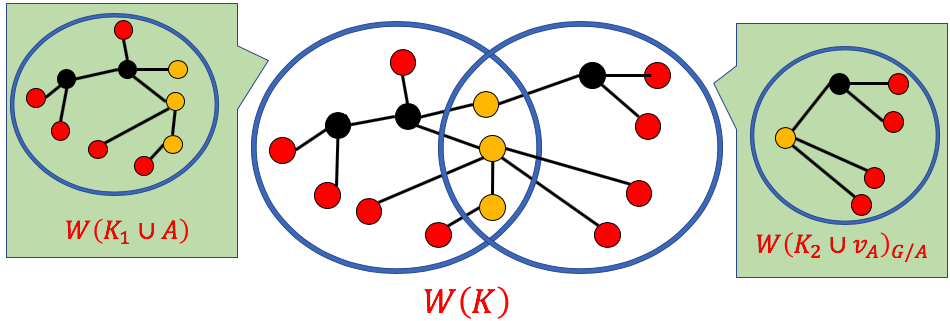
| Abstract | Minimum Steiner tree problem is a well-known NP-hard problem. For the minimum Steiner tree problem in graphs with $n$ vertices and $k$ terminals, there are many classical algorithms that take exponential time in $k$. In this paper, to the best of our knowledge, we propose the first quantum algorithm for the minimum Steiner tree problem. The complexity of our algorithm is $\mathcal{O}^*(1.812^k)$. A key to realize the proposed method is how to reduce the computational time of dynamic programming by using a quantum algorithm because existing classical (non-quantum) algorithms in the problem rely on dynamic programming. Fortunately, dynamic programming is realized by a quantum algorithm for the travelling salesman problem, in which Grover's quantum search algorithm is introduced. However, due to difference between their problem and our problem to be solved, recursions are different. Hence, we cannot apply their technique to the minimum Steiner tree problem in that shape. We solve this issue by introducing a decomposition of a graph proposed by Fuchs et al. |
- Note
Full version of the paper is available at <a href="https://arxiv.org/abs/1904.03581">https://arxiv.org/abs/1904.03581</a> - Following files are available.
- Entry for BibTeX
@InProceedings{Miyamoto2019, author = {Masayuki Miyamoto and Masakazu Iwamura and Koichi Kise}, title = {A Quantum Algorithm for Minimum Steiner Tree Problem}, booktitle = {Proc. 19th Asian Quantum Information Science Conference (AQIS2019)}, year = 2019, month = aug, numpages = {2}, location = {Seoul, Korea} }